| Columns Retired Columns & Blogs |
but hifi news has been doing it for ages,along with the very revealing frequency matching for loudspeaker pairs,which shows
the consistency of the workmanship you're paying for.
Doing it in silicon
There are two obvious ways to go about determining device dissipation with music program. First, we could simultaneously measure voltage across and current through either the loudspeaker or the output device—at an output level that will not cause the amplifier protection to operate—and directly calculate instantaneous device dissipation. This is what Benjamin did in his work. Alternatively, we can use a computer-simulation approach, which has various advantages. We can obtain a result faster than in real time (provided we use FFT convolution), and we can assess any speaker for which we have impedance data, even though that speaker is no longer to hand. (The latter is an attractive benefit; the review samples of all three speakers whose EPDR I've plotted were returned many moons ago.)
How we go about this simulation is to design a digital filter that will convert the signal voltage across the speaker into the signal current through it, so that we generate the same data as we would by measurement. To design this filter, we first have to calculate the inverse of the speaker's complex impedance—ie, its admittance—because voltage times admittance gives us the current. This frequency-dependent, complex admittance can then be converted into an FIR digital filter by applying the inverse fast Fourier transform (IFFT) to take us from the frequency domain to the time domain. The resulting filter is then applied, by convolution, to the input voltage (represented by the sample values in a WAV file) to generate the current waveform. The output-device dissipation can then be calculated sample by sample, and the result analyzed to see whether it is as high on music signals as our analysis of the impedance data suggests.
This process isn't quite so straightforward as just described because the sampling rate used to obtain the impedance data will generally not be the same as that of the WAV files we wish to process, so an adjustment must be made. In the case of the data used here—MLSSA impedance data measured using DRA Labs' supplied high-resolution setup script—the sampling rate is 65.57kHz and the output file contains 9995 data points from 2Hz to 20kHz. In order to use this to design an admittance filter, I extracted the first 8192 data points from the modulus and phase files, interpolated the 0Hz modulus, set the 0Hz phase to 0°, and then used these 8193 points to generate a 16,384-point filter. (We're not concerned with filter efficiency here, only accuracy.) To give the correct result, this filter was then applied to WAV files that had first been downsampled to (65.57kHz ˜ 2 =) 32,785Hz. As a result, the maximum signal frequency in the simulation is not 20kHz, as in the data, but 16.4kHz.
It's no accident that I've chosen as examples here three speakers whose EPDR behavior is significantly different. The JBL's EPDR dips to its minimum at low frequency; the B&W has prominent dips at both low and midrange frequencies, associated with bass and midrange system resonances, with a third, shallower dip associated with the tweeter resonance; while the Final's EPDR bottoms out at very high frequency, but also has a significant dip around 300Hz. Bearing in mind the typical spectrum of music, we might reasonably expect the B&W to present the most challenging amplifier load in practice, followed perhaps by the Final and then the JBL.
Four diverse music excerpts were chosen for the analysis, not entirely at random. I didn't have the luxury, as Eric Benjamin did, of assessing a large number of CDs and cherry-picking, so I selected source material with an eye to the EPDR results, hoping to choose recordings that would result in something like worst-case dissipation. Three were extracts from single-instrument recordings on the European Broadcasting Union's SQAM (sound quality assessment material) test disc, of flute, triangle, and soprano voice. The flute and soprano pieces have strong spectral content around 700Hz, which I hoped would probe the B&W 802D's midrange EPDR dip, while the triangle item has energetic HF to provoke the Final 600i. The fourth item—track 3 of Brian Bromberg's Wood (A440 Music 4001), a string-bass solo take on Lennon and McCartney's "Come Together"—was chosen for having strong midbass fundamentals where the 802D and JBL 1400 Array both have their lowest EPDRs.
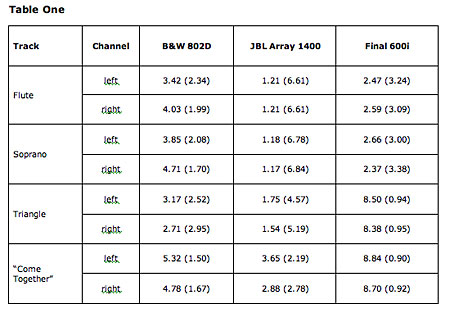
Table 1 lists the highest device dissipations recorded for each channel of each track on each speaker, taking the worst-case dissipation into an 8 ohm resistance as the baseline. The second figure in brackets in each case is the equivalent EPDR. All the values fall within the ranges shown in figs. 5–7 and 8–10, and mostly they follow the expected pattern, the one surprise being the high values recorded for the Brian Bromberg track and the Final 600i. Taken together, these figures confirm that the orders of EPDR identified in figs. 8–10 are of real, practical significance when playing music signals: speakers really can make these high demands of amplifier output-device dissipation in normal use. If the amplifier's protection is invoked as a result, then its output will be clipped, even though the speaker's voltage and current demands may be within its capability.
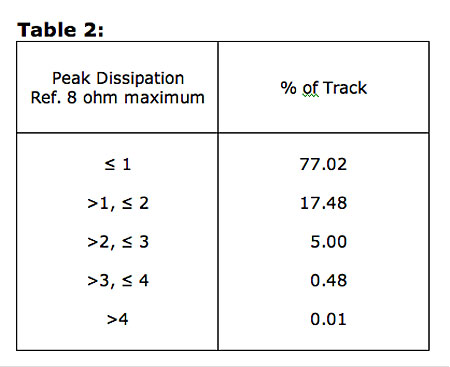
How frequently do these extreme dissipation events occur? Some insight into this is given in Table 2, which shows the proportion of time that the dissipation factor fell within particular bounds for the B&W 802D on the left channel of the Bromberg track. For 5% of the time the speaker's EPDR is less than 4 ohms, and for 0.48% of the time below 2.7 ohms. So this is clearly a potentially significant effect with difficult source material. It's also obvious from these results why the B&W 802D has a reputation for being an amplifier ball-breaker.
Heard before
All in all, there's little new here that Eric Benjamin's work didn't reveal 13 years ago. The EPDR concept is useful, I think. So is the simulation approach using digital filtering, since it allows results to be obtained more quickly, and with nothing more than conventional impedance modulus and phase measurements by way of input. But no excuse is necessary for reprising Benjamin's work, because its import seems not to have suffused audiophile consciousness. Speaker reviews don't address this issue, and neither do many speaker manufacturers, who are apparently happy to throw the output-device dissipation problem over the fence for amplifier designers to deal with. Jim Lesurf, who recently wrote about this issue for Hi-Fi News (May 2007, pp.100–102), jokingly postulated the existence of SCAMP—the Society for Cruelty to Amplifiers. If it existed, its membership would be thriving.

That this article was written in 2007, by Keith Howard, who coined the term, and who until recently was doing the loudspeaker measurements at HFN. Stereophile has now posted online somewhat more than half its historical archives; we post more historical articles each month.
Jim Austin, Editor
Stereophile