| Columns Retired Columns & Blogs |
Building the Hi-Fi House Page 2
A Matter of Modes
Chapter 11 of F. Alton Everest's The Master Handbook of Acoustics (Tab Books) proved quite useful in determining optimal room dimensions. I settled on a 1.0:1.6:2.33 (H:W:D) room ratio. A 10' ceiling would mean a 16'-wide room---good, but a little less than what I wanted. An 11' ceiling resulted in a 17.6'-wide by 25.6'-long room. I cheated a bit and rounded the last two dimensions up to the nearest foot, for overall room dimensions of 11' high by 18' wide by 26' long (fig. 1).
Footnote 1: Your homework is to figure out where this number came from. The velocity of sound equals frequency times wavelength; the velocity of sound at sea level is about 1130ft/s. Standing waves build up where a half cycle of sound at a given frequency, or multiples of it, fits into a given space. Got it now? If you don't, don't worry. You don't need to understand the derivation to do the math.
Chapter 11 of F. Alton Everest's The Master Handbook of Acoustics (Tab Books) proved quite useful in determining optimal room dimensions. I settled on a 1.0:1.6:2.33 (H:W:D) room ratio. A 10' ceiling would mean a 16'-wide room---good, but a little less than what I wanted. An 11' ceiling resulted in a 17.6'-wide by 25.6'-long room. I cheated a bit and rounded the last two dimensions up to the nearest foot, for overall room dimensions of 11' high by 18' wide by 26' long (fig. 1).
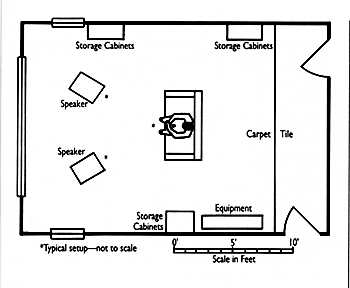
Fig.1 TJN's dedicated listening room.
Room modes result from a buildup of sound pressure within a room at the frequencies that are functions of the room's dimensions. Modes cause problems primarily in the bass---below about 250Hz. They also exist at higher frequencies, but only in the bass are they isolated enough to cause significant audible aberrations. There are three main types of room modes: axial, tangential, and radial. Of the three, the axial modes---those occurring between opposite walls---are the strongest and most potentially troublesome.
Calculating the axial modes for a given rectangular room is simple, though a little tedious: simply divide 565 (footnote 1) by the room length, then repeat for the width and height. Next, list all of the possible whole-integer multiples of these frequencies, up to about 250Hz. Put all of these frequencies in ascending order, and voila!, you have a list of your room's axial modes. Ideally, they should be more or less evenly distributed by frequency. This has never happened in my experience, with any real listening room of less than baronial size.
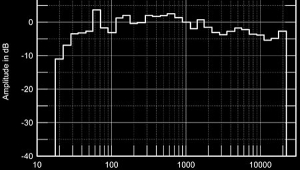
But it's best to avoid a pileup of modes at a specific frequency, or two very closely spaced frequencies. For the room dimensions I chose, there were no pileups up to 250Hz (fig.2), though two modes in the midbass are spaced by 2Hz (64Hz and 66Hz). There are other close modal spacings around 150Hz, 200Hz, and 205Hz. Calculating the modes for a number of other suitable room dimensions, however, revealed that no combinations were noticeably better than this, and many were far worse. There are no perfect dimensional ratios for a small room---and even fairly large domestic rooms such as this one are "small" relative to bass wavelengths; there's only good, bad, and worse. The dimensions I picked held promise of being among the good ones.
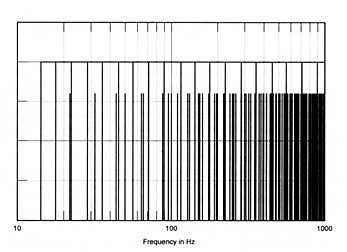
Fig.2 Density of calculated axial room modes in TJN's room, grouped into one-third-octave bands (up to 1 kHz). Note the relative sparseness of room modes at low frequencies.
Long after my house dimensions were in, ah, concrete, an interesting three-part article appeared in Speaker Builder magazine on building a listening room: "What Makes Your Room Hi-Fi," by Joseph Saluzzi, in issues Six/'92, One/'93, and Two/'93. In the first of these, Saluzzi also tries to determine optimal dimensions for a listening room.
His nephew developed a far more efficient method than I had available---a computer program which calculates the modes and the standard deviation between them, recalculates the modes for every 1" increment of dimensional change, and rejects dimensions with mode pileups. A less elaborate program, "Modes for your Abodes," which prompts the user to input room dimensions and calculates and sorts the modes by frequency or mode type, is available through Old Colony Sound Laboratory. (footnote 2) I haven't tried it---my building days are over for now, thank you very much, but it could save you considerable work and tedium when trying to zero-in on desirable room dimensions. You could also use it to analyze your existing room.
Cathedral Ceilings
All of the methods I'm aware of for analyzing the modes of a prospective or existing room assume a closed, rectangular space with rigid walls. A sloped ceiling complicates the calculations; you'll still be able to calculate the modes for the room's length and width, but what do you do about the height? I have never seen this addressed in the references available to me, but it would seem intuitive that, instead of discrete modes related to a specific ceiling height and multiples of this mode, you'll have an infinite number of much smaller, probably negligible modes distributed according to the variation of ceiling height, running from the minimum to the maximum height.
One disadvantage here is that there will now be no strong, distinct "height" modes to help fill in and smooth out the width and length modes. Since modes can't be eliminated, the only other alternative is to spread them out as evenly as possible. "Gaps" in the modal coverage cause the remaining modes to stick out more. With a cathedral ceiling, the width and length modes could result in the room having a spottier bass response than it would have with a flat ceiling. A sloped ceiling will, however, improve the diffusion characteristics of the listening room, "opening up" the sound. I've enjoyed music in rooms that have one-way sloped ceilings that work quite well, but I avoided this design for my room because of its essentially unpredictable results. By the way, when you get to the popular "open plan" living space, there's no reliable way to predict the result.
The result of all these philosophical and mathematical thoughts was plain: the dedicated listening room at Casa Norton would be rectangular with a high, flat ceiling.
Footnote 1: Your homework is to figure out where this number came from. The velocity of sound equals frequency times wavelength; the velocity of sound at sea level is about 1130ft/s. Standing waves build up where a half cycle of sound at a given frequency, or multiples of it, fits into a given space. Got it now? If you don't, don't worry. You don't need to understand the derivation to do the math.
Footnote 2: P.O. Box 243, Peterborough, NH 03458-0243. Tel: (603) 924-6371.
- Log in or register to post comments