| Columns Retired Columns & Blogs |
(Correct me as necessary...)
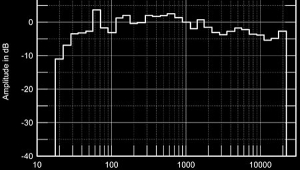
The "low-pass filter" in JA's above discussion is not the "oversampling digital filter" (which is, in all reality, optional) nor is it the SAME as the output low-pass filter (e.g., analog, multi-pole).
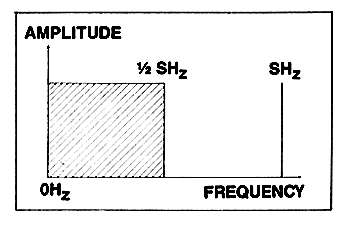
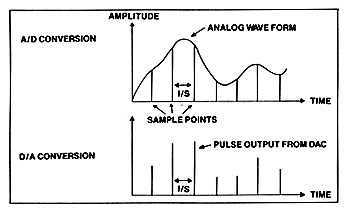
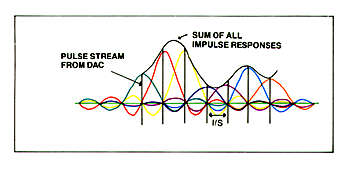
Rather, the "low-pass filter" in JA's above discussion, is a mathematical (on-paper, or theoretical) concept of digital-to-analog RECONSTRUCTION.
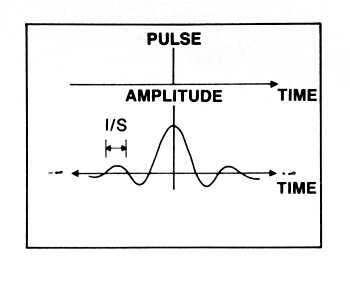
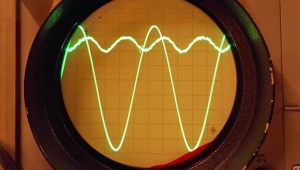
To put it plainly, if all you had was a bare-bones DAC chip (take one of the first-generation CD players with a chip like Philips TDA1540) -- so, no oversampling -- the above discussion of "low-pass filter", and (sin x)/x curve and impulse response would STILL apply.
I think the confusion comes from the rather liberal way the term "reconstruction filter" is used. I.e., sometimes used as an alternate to oversampling (e.g, 4x, or 8x) ... as well as the textbook terminology (as JA notes above), or here...
http://en.wikipedia.org/wiki/Reconstruction_filter
(Wiki seems to suggest that the RF can ALSO be the output analog filter, e.g., brick-wall, multi-pole, etc. AFTER the DAC chip)
Again I might stand well corrected!!