| Columns Retired Columns & Blogs |
Lamm ML2.1 monoblock power amplifier Measurements
Sidebar 3: Measurements
Following Vladimir Lamm's instructions, I let the ML2.1 run for an hour or so before I checked the output tube's plate voltage and current. While the voltage was correct at 175V DC, the current was a little high at 0.35A. I adjusted it to read exactly 310mA, as recommended in the excellent owner's manual (one of the best I have encountered). All measurements were made using the unbalanced RCA input, as the XLR is provided as a convenience only. (Pin 2 parallels the RCA jack, pin 3 is connected to ground.)
As expected, the ML2.1's voltage gain dropped as the nominal value of the output transformer halved. From the 16 ohm tap driving 16 ohms, gain was a healthy 27.4dB. This dropped to 24.25dB from the 8 ohm tap into 8 ohms, and 20.9dB from the 4 ohm tap into 4 ohms. Absolute polarity was correct from all three outputs. The input impedance measured 41k ohms at 20Hz and 39.3k ohms at 1kHz. While it dropped to 23k ohms at 20kHz, this will not be significant.
Considering that the amplifier is single-ended, uses a single output tube, and has very little overall negative feedback, its output impedance was relatively low, at 2 ohms (16 ohm tap), 1.2 ohms (8 ohm tap), and 0.8 ohm (4 ohm tap). These figures held from the low bass through the mid-treble; the source impedance rose slightly at the top of the audioband, to 2.4 ohms, 1.8 ohms, and 0.9 ohm, from the 16, 8, and 4 ohm taps, respectively. As a result of this fairly low output impedance, the frequency-response variation into our standard simulated loudspeaker was also fairly low, ranging from ±0.5dB from the 4 ohm tap (fig.1) to ±1dB from the 16 ohm tap (fig.3).
Again considering what kind of amplifier this is, the ML2.1 has an extraordinarily wide frequency response, especially from the 4 ohm tap into higher impedance loads, where the small-signal output was just 1dB down at 95kHz into 16 ohms (fig.1). The bandwidth decreased into lower impedances, but the upper-frequency response from the Lamm's 4 ohm tap into 2 ohms was still -1dB at 29kHz and just -0.2dB at 20kHz. The 8 ohm tap has slightly less high-frequency output (fig.2), but is still flat to the audioband limit of 20kHz into higher impedances. The response from the 16 ohm tap starts to roll off in the top audio octave into loads of 4 ohms and below (fig.3), but this will not be of practical relevance.
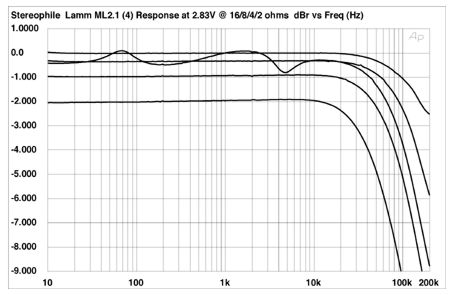
Fig.1 Lamm ML2.1, 4 ohm tap, frequency response at 2.83V into (from top to bottom at 2kHz): simulated loudspeaker load, 16 ohms, 8 ohms, 4 ohms, 2 ohms (0.5dB/vertical div.).
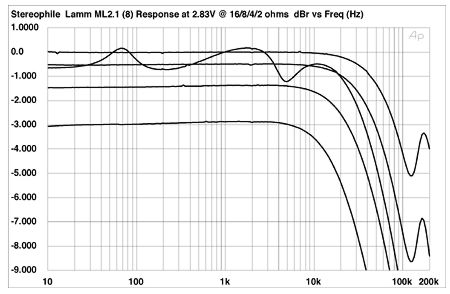
Fig.2 Lamm ML2.1, 8 ohm tap, frequency response at 2.83V into (from top to bottom at 2kHz): simulated loudspeaker load, 16 ohms, 8 ohms, 4 ohms, 2 ohms (0.5dB/vertical div.).
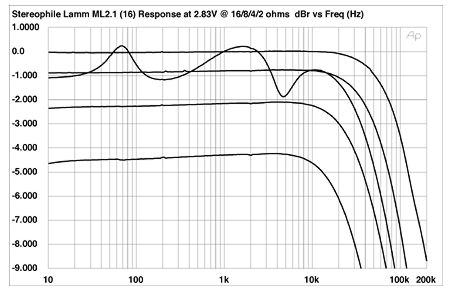
Fig.3 Lamm ML2.1, 16 ohm tap, frequency response at 2.83V into (from top to bottom at 2kHz): simulated loudspeaker load, 16 ohms, 8 ohms, 4 ohms, 2 ohms (0.5dB/vertical div.).
However, note that an ultrasonic resonance makes an appearance in this graph. Lying at an extraordinarily high 172kHz, this is a tribute to the maker of Lamm's output transformers—and because it is well-damped, I doubt that it will affect sound quality. This resonance was absent from the 16 ohm family of responses, though a slight upward tilt to the response (+0.5dB, 20Hz-5kHz) was evident with this tap driving 2 ohms. As the amplifier's output would be very distorted under these conditions, this slight frequency imbalance would be the least of the problems.
Note that the Lamm's low-frequency response is commendably flat down to the 10Hz limit of these graphs, which in turn means that the amplifier's reproduction of a 1kHz squarewave is almost perfectly square (fig.4). The 10kHz squarewave response is also superb, though a very slight amount of ultrasonic ringing can be just discerned on the leading edges (fig.5), correlating with the damped resonance mentioned above.

Fig.4 Lamm ML2.1, 8 ohm tap, small-signal 1kHz squarewave into 8 ohms.
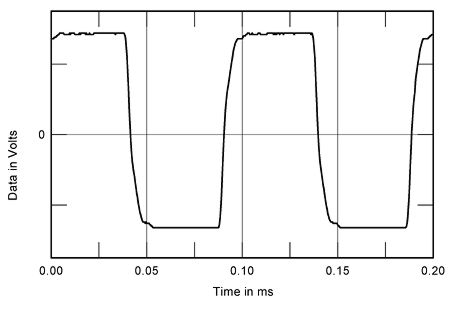
Fig.5 Lamm ML2.1, 8 ohm tap, small-signal 10kHz squarewave into 8 ohms.
The ML2.1 is very quiet, with a measured unweighted, wideband signal/noise ratio of 86dB ref. 1W into 8 ohms from the 8 ohm tap, this figure increasing to an excellent 105dB when A-weighted. However, the amplifier showed some sensitivity to grounding arrangements; I had to experiment to get the lowest noise, with some ultrasonic noise appearing when the grounding between the amplifier and my test equipment wasn't optimal.
Lamm specifies the ML2.1 as giving a maximum output of 18W from each of the three transformer taps at 3% distortion. Figs.6, 7, and 8 show how the percentage of distortion and noise in the amplifier's output changes with increasing output power and decreasing load impedance, from the 4, 8, and 16 ohm output taps, respectively. While the single-ended design topology and minimal use of negative feedback results in steadily increasingly nonlinear behavior with increasing power, the ML2.1 actually gives out more power, and sometimes at lower levels of distortion, than specified. Refreshing. Note, however, that the THD drops below 0.1% only at low levels, when the load is higher than the nominal value of the tap.
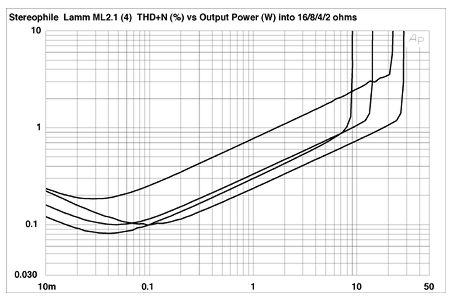
Fig.6 Lamm ML2.1, 4 ohm tap, distortion (%) vs 1kHz continuous output power into (from bottom to top at 1W): 8 ohms, 16 ohms, 4 ohms, 2 ohms.
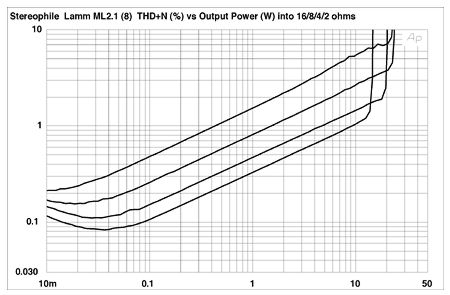
Fig.7 Halcro dm38, 1kHz waveform at 120W into 4 ohms (top), 0.0055% THD+N; distortion and noise waveform with fundamental notched out (bottom, not to scale).
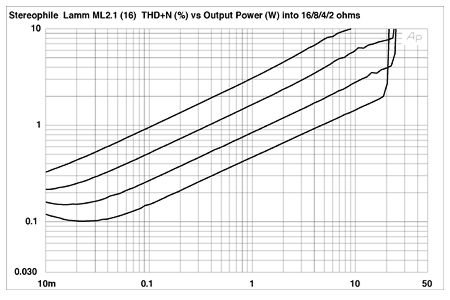
Fig.8 Lamm ML2.1, 16 ohm tap, distortion (%) vs 1kHz continuous output power into (from bottom to top at 1W): 16 ohms, 8 ohms, 4 ohms, 2 ohms.
The most power is available when the tap is matched to the load, with 19.4W available into 4 ohms from the 4 ohm tap, 12.5W into 8 ohms from the 8 ohm tap, and 5.2W into 16 ohms from the 16 ohm tap. All these figures are at 1% THD, our usual definition of "clipping." However, the waveform wasn't actually clipped on the oscilloscope screen at these levels; relaxing the definition to Lamm's specified 3% THD gave 28W into 4 ohms, 19.9W into 8 ohms, and 20.4W into 16 ohms, from the appropriate taps.
Figs.9, 10, and 11 show how the ML2.1's small-signal THD+noise percentage varies with frequency and load. As expected from the output power graphs, there is more distortion apparent than I would like to see in absolute terms, especially into loads much lower than the output tap value. However, the distortion is predominantly second-harmonic in nature (fig.12), which will work against the distortion being a) audible and b) objectionable.
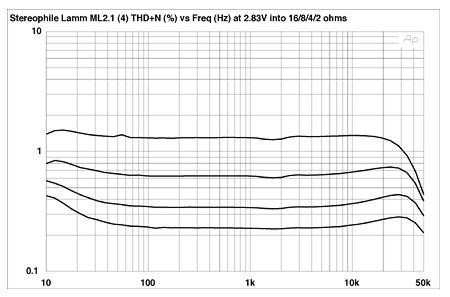
Fig.9 Lamm ML2.1, 4 ohm tap, THD+N (%) vs frequency at 2.83V into (from bottom to top at 1W): 16 ohms, 8 ohms, 4 ohms, 2 ohms.
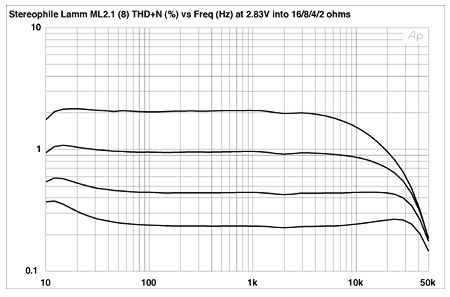
Fig.10 Lamm ML2.1, 8 ohm tap, THD+N (%) vs frequency at 2.83V into (from bottom to top at 1W): 16 ohms, 8 ohms, 4 ohms, 2 ohms.
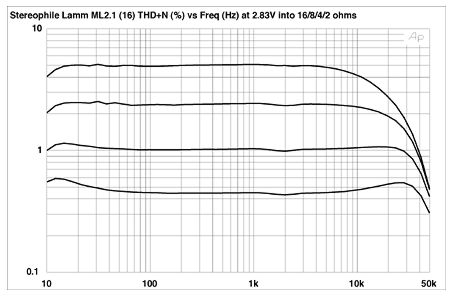
Fig.11 Lamm ML2.1, 16 ohm tap, THD+N (%) vs frequency at 2.83V into (from bottom to top at 1W): 16 ohms, 8 ohms, 4 ohms, 2 ohms.
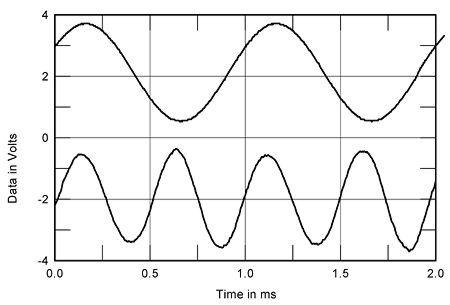
Fig.12 Lamm ML2.1, 4 ohm tap, 1kHz waveform at 2W into 4 ohms (top), 0.5% THD+N; distortion and noise waveform with fundamental notched out (bottom, not to scale).
However, and perhaps more important, the Lamm's distortion spectrum doesn't vary with frequency or with output tap. Fig.13 shows the spectrum of the amplifier's output as it drives a 50Hz sinewave at 1W into 8 ohms, while fig.14 shows the spectrum of a 1kHz sinewave driven at the same level into 4 ohms from the 4 ohm tap. They are very similar, with the second harmonic by far the highest in level. The third harmonic lies much lower in level—more so with the low-frequency tone than with the midrange tone—and power-supply-related spuriae are better than 100dB down, which is excellent.
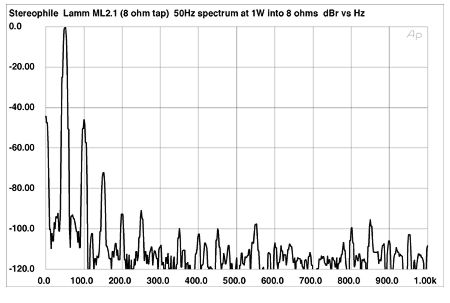
Fig.13 Lamm ML2.1, 8 ohm tap, spectrum of 50Hz sinewave, DC-1kHz, at 1W into 8 ohms (linear frequency scale).
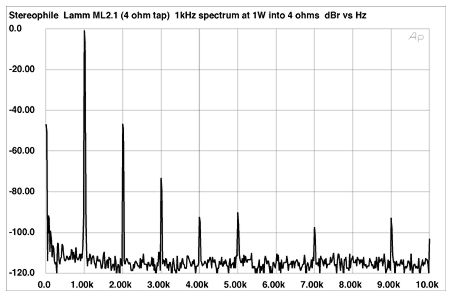
Fig.14 Lamm ML2.1, 4 ohm tap, spectrum of 1kHz sinewave, DC-10kHz, at 1W into 4 ohms (linear frequency scale).
Whether or not an amplifier's primarily second-harmonic distortion is subjectively benign (even if audible) depends on there not being high levels of intermodulation distortion. Fig.15 shows the spectrum of the ML2.1's output while it drove an equal mix of 19kHz and 20kHz tones at 2W into 8 ohms from the 8 ohm tap. The higher-order components are 80dB down, though the 1kHz difference tone lies at -52dB (0.25%), a little higher than I would have wished.
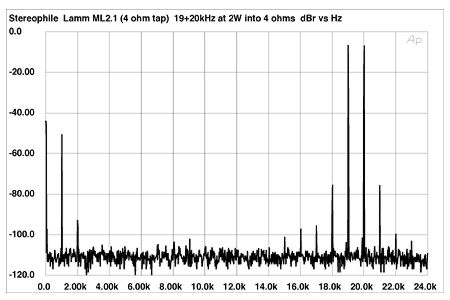
Fig.15 Lamm ML2.1, 8 ohm tap, HF intermodulation spectrum, DC-24kHz, 19+20kHz at 2W into 8 ohms (linear frequency scale).
Yes, the ML2.1's basic linearity is not anywhere as near what is routinely achieved by high-feedback, solid-state amplifier designs. But this is almost entirely due to the fact that the Lamm's output stage is single-ended. Considering that the circuit uses very little overall negative feedback, its measured performance suggests that the amplifier circuit's open-loop performance is actually very linear. In its idiosyncratic way, the ML2.1 is good engineering.—John Atkinson
- Log in or register to post comments



































