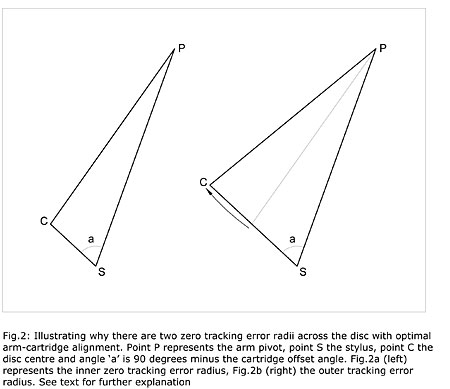
| Columns Retired Columns & Blogs |
Hi there,
Thanks for this very interesting explanation.
There are 2 things though:
It is stated that it would be good to have a alignment tool delivered with cartridges that obviously is referring to the cartridge body:
I have found that cantilevers and styluses are never 100% aligned correctly, so how to deal with this?
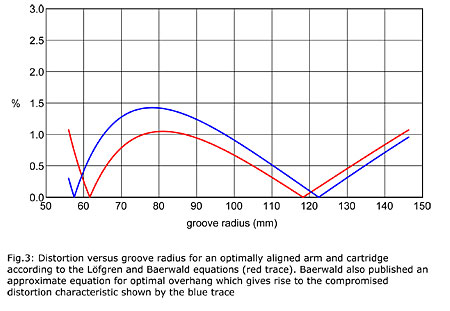
The other thing is the Lofgren B method on which you comment that the approach does not make sense to you because of, as I read it, the higher distortion at the end of the disc.
If I look at my records, most of them don't have modulated grooves there where Lofgren B is exceeding the distortion of the max mid area distortion of the Bearwald, so therefore I think that Lofgren B is the better one...(for me at least)